Computed Design Power

Computed Design Power

p1
=2100
p2
=110.526
Gear Speed

G
= n
p
(

G1
= 2100(

)
G2
= 110.526(

G1
= 110.526
G2
= 98
Train Value (Input Speed & Nominal Output Speed)
TV =

TV=

TV= 21.43




Gear Set 1 Gear Set 2
Velocity Ratio
VR
1
=

VR
1
=

VR
2
=

VR
1
= 19 VR
2
= 1.12

Number of Gear Teeth
N
G1
= N
P1
(VR
1
) N
G2
=N
P2
(VR
2
)
N
G1
= 10(19) N
G2
= 47(1.12)
N
G1
= 190 N
G2
= 53
Actual Velocity Ratio
VR
1
=

VR
2
=

VR
1
=

VR
2
=

VR
1
= 19 VR
2
= 1.12
Actual Gear Speed

G
=
p
(

G
= 110.526(

G
= 98.01

Train Value (Input Speed & Actual Output Speed)
TV =

TV =

TV = 21.42
Pinion Diameters
D
P1
=

D
P2
=

D
P1
=

D
P2
=

D
P1
= .625 D
P2
= 5.875
Gear Diameters
D
G1
=

D
G2
=

D
G1
=

D
G2
=

D
G1
= 12 D
G2
= 6.625
Center Distance
C =

C
1
=

C
2
=

C
1
=6.25 C
2
=6.25
Pitch Line Speed
V
t
=

V
t1
=

V
t2
=

V
t1
= 343.612 V
t2
= 2039.96
Dynamic Factor
B =

B =

B = .396
A= 50+56(1.0-.396)
A= 83.8
K
V
=

K
V1
=

K
V2
=

K
V1
= 1.082 K
V2
= 1.187
Transmitted Load
W
t
=

W
t1
=

W
t2
=

W
t1
= 2400.96 W
t2
= 404.42
Face Limit Values
Upper: F
1
=

= 1 Upper: F
2
=

= 2.0
Lower: F
1
=

= .50 Lower: F
2
=

= 1.0
Nominal: F
1
=

= .75 Nominal: F
2
=

= 1.5
Size Factor
K
s
=1
Elastic Coefficient for Steel
C
p
=2300
Quality Number
Q
V
=10 Q
V
=10
Geometry Factor
J
p1
=0.38 J
p2
= 0.38
J
G1
=0.43 J
G2
=0.41



Pitting Resistance for Geometry Factor Calculation
C
1
=

C
1
=

C
1
=

C
1
=

C
1
= 4.564 C
1
= 8.037
C
2
=

C
2
=

C
2
=

C
2
=

C
2
= 86.729 C
2
= 48.386
C
3
= C
3
=
C
3
= 2.952 C
3
= 2.952
C
4
= 0.5*

+ C
4
= 0.5 *

+
C
4
= 0.5 *

+ C
4
= 0.5 *

+
C
4
= 5.55 C
4
= 2.57
C
x
=

C
x
=

C
x
=

C
x
=

C
x
= 1.5222 C
x
= 0.9599
C
c
=

C
c
=

C
c
=

C
c
=

C
c
= 0.15266 C
c
= 0.08404
I =

I = I =

Load Distribution Factor
C
pf
=

C
pf
=

C
pf
=

C
pf
=

C
pf
= 0.091875 C
pf
= 0.00678
C
ma
= 0.127+0.0158F-1.093E
-4
F
2
C
ma
= 0.127+0.0158F-1.093E
-4
F
2
C
ma
= 0.127+0.0158(.75) -1.093E
-4
(.75)
2
C
ma
= 0.127+0.0158(1.5) -1.093E
-4
(1.5)
2

C
ma
=0.138789 C
ma
= 0.150454
K
m
=1+C
pf
+C
ma
K
m
=1+0.091875+0.138789 K
m
=1+0.0615+0.15045

K
m
= 1.23 K
m
= 1.157
Bending Stress Number
S
tp
=

S
tG
= S
tp
(

)
S
tp
=

S
tp
=

S
tp
= 358.9 kpsi S
tp
= 166.9 kpsi
S
tG
=

S
tG
=

S
tG
= 316.3 kpsi S
tG
= 154.6 kpsi
Adjusted Allowable Bending Stress Numbers
S
atp
> S
tp

S
atp
=

S
atp
=

S
atp
= 389.4 kpsi S
atp
= 175.1 kpsi
S
atG
=

S
atG
=

S
atG
= 334.5 kpsi S
atG
= 162.2 kpsi

Contact Stress Number
S
c
= C
p

S
c
= 2300

S
c
= 2300

S
c
= 557.3 kpsi S
c
= 297.4 kpsi
Adjusted Contact Stresses
S
acp
> S
cp

S
acp
=

S
acp
=

S
acp
=632.7 kpsi S
acp
= 327.04 kpsi
S
acG
> S
cG

S
acG
=

S
acG
=

S
acG
= 612.8 kpsi S
acG
= 323.7 kpsi










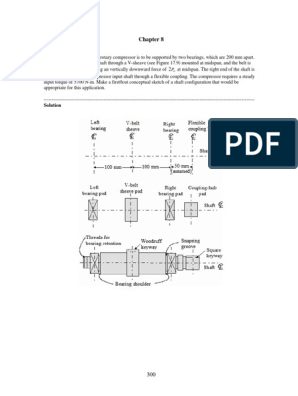
Appendix 2: Shafts 1-3 Design Calculations
Shaft Design Bending Moment and Shear force Calculation
S

n
= S
n
C
s
C
R
= (42000)(0.75)(0.81) = 25515psi
S
n
= 42000 psi
S
y
= 83000 psi
S
u
= 118000 psi
Shaft #1 Shaft#2
T (input) =

T (input) =

T (input) = 750 lbf in T (input) = 14250 lbf in
Shaft #3
T (input) =

T (input) = 16071.4 lbf in

Shaft 1: Tangential & Radial Forces
W
t
=

= 2400 lbf
W
r
= W
t
(tan(20
o
)) = (2400)(tan(20
o
)) = 873.529 lbf
Shaft 1: Tangential and Radial Reaction Forces
(Tangential)
+ F
x
= 0
= -R
Ax
R
Cx
+ W
t

R
Cx
= 2400 - R
Ax



Counter Clockwise (+)
M
Rcx
= 0
= R
Ax
(3.0in) W
t
(1.5in)
R
Ax
=

= 1200 lbf
R
Cx
= 2400 1200 = 1200 lbf
(Radial)
+ F
y
= 0
R
Cy
=873.529 - R
Ay

Counter Clockwise (+)
M
Rcy
= 0
= R
Ay
(3.0in)- W
r
(1.5in)
R
Ay
=

= 436.76 lbf
R
Cy
= 873.529 436.76 = 436.76 lbf
Tangential Shear & Bending Moment Calculations
From A to B:
+ F
x
= 0;
-R
Ax
V = 0
V = -R
Ax
= -1200 lbf
M
o
= 0
R
Ax
(x) + M = 0
M = -R
Ax
(x)
M = -1200(x)
From B to C:
+ F
y
= 0;
-R
Ax
V + W
t
= 0
V= -1200 + 2400
V = 1200 lbf
M
o
= 0
M + R
Ax
(x) W
t
(x-1.5) = 0
M = -R
Ax
(x) +W
t
(x-1.5)
M = -1200(x) + 2400(x) 750
M = 1200(x) - 750
Radial Shear & Bending Moment Calculations
From A to B:
+ F
y
= 0;
-R
Ax
V = 0
V = -R
Ax
= -436.76 lbf
M
o
= 0
R
Ax
(x) + M = 0
M = -R
Ay
(x)
M = -436.76(x)
From B to C:
+ F
y
= 0;
-R
Ay
V + W
t
= 0
V= -436.76 + 2400
V = 1963.24 lbf
M
o
= 0
M + R
Ay
(x) W
r
(x-1.5) = 0
M = -R
Ay
(x) +W
r
(x-1.5)
M = -436.76(x) + 873.52(x) 1310.28
M = 436.76(x) 1310.28

Shaft 2: Tangential & Radial Forces
W
t1
=

= 2400 lbf
W
r1
= W
t1
(tan(20
o
)) = (2400)(tan(20
o
)) = 873.52 lbf
W
t2
=

= 4851.06 lbf
W
r2
= W
t2
(tan(20
o
)) = (4851.06)(tan(20
o
)) = 1765.64 lbf
Shaft 2: Tangential and Radial Reaction Forces
(Radials)
+ F
x
= 0
= R
Ax
W
t1
- W
t2
+ R
Dx

R
Dx
=- R
Ax
+ 2400+4851.06
R
Dx
= 7251.06 -R
Ax

Counter Clockwise (+)
M
RDx
= 0
=- R
Ax
(8.5) + Wr
1
( 7.0in) + W
r2
(2.0in)
R
Ax
=

= 2391.92 lbf
R
Cx
= 7251.06 2391.92 = 4859.14 lbf
(Tangentials)
+ F
y
= 0
= R
Ay
W
r1
+ W
r2
+R
Dy

R
Dy
= 873.52 1765.64 - R
Ay
R
Dy
= -892.12 - R
Ay
Counter Clockwise (+)
M
Rcy
= 0
= -R
Ax
(8.5in)+ W
t1
(7.0in)-W
t2
(2.0in)
R
Ay
=

= 835.045 lbf
R
Cy
= -892.12 835.045 = -1727.16 lbf
Tangential Shear & Bending Calculations
From A to B:
+ F
x
= 0;
R
Ax
V = 0
V = R
Ax
= 835.045 lbf
M
o
= 0
-R
Ax
(x) + M = 0
M = R
Ax
(x)
M = 835.045 (x)
From B to C:
+ F
x
= 0;
R
Ax
V - W
t1
= 0
V= 835.045 - 2400
V = -1564.96 lbf
M
o
= 0
M - R
Ax
(x) + W
t1
(x-1.5) = 0
M = +R
Ax
(x) -W
t1
(x-1.5)
M = +835.045(x) - 2400(x) + 3600
M = -1564.96(x) +3600
From C to D:
+ F
x
= 0;
R
Ax
-V- W
t1
+ W
t2
= 0
V = 835.045 - 2400 + 4851.06
V = 3286.11 lbf
M
o
= 0
M - R
Ax
(x) + W
t1
(x-1.5) W
t2
(x-6.5) = 0
M = R
Ax
(x) -W
t1
(x-1.5) +W
t2
(x-6.5)
M = 835.045(x) - 2400(x) +4851.06(x)+3600-161532
M = 3286.11(x) - 157932
Radial Shear & Bending Moment Calculations
From A to B:
+ F
y
= 0;
R
Ay
V = 0
V = R
Ay
= 2391.92 lbf
M
o
= 0
-R
Ay
(x) + M = 0
M = R
Ay
(x)
M = 2391.92 (x)
From B to C:
+ F
y
= 0;
R
Ay
V W
r1
= 0
V= 2391.92 - 873.52
V = 1518.4 lbf
M
o
= 0
M - R
Ay
(x) + W
r1
(x-1.5) = 0
M = R
Ay
(x) -W
r1
(x-1.5)
M = 2391.92 (x) - 873.52 (x) 1310.28
M = 1518.4 (x) 1310.28
From C to D:
+ F
y
= 0;
R
Ay
V- W
r1
W
r2
= 0
V = 2391.92 - 873.52 - 1765.64
V = -247.24 lbf
M
o
= 0
M - R
Ay
(x) + W
r1
(x-1.5) +W
r2
(x-6.5) = 0
M = R
Ay
(x) -W
r1
(x-1.5) W
r2
(x-6.5)
M = 2391.92 (x) - 873.52 (x) 1765.64 (x) + 1310.28 + 11476.7
M = -247.24 (x)+12787
Shaft 3: Tangential & Radial Forces
W
t
=

= 4851.74 lbf
W
r
= W
t
(tan(20
o
)) = (4851.74)(tan(20
o
)) = 1765.89 lbf
Shaft 3: Tangential and Radial Reaction Forces
(Tangential)
+ F
x
= 0
= -R
Ax
R
Cx
+ W
t

R
Cx
= 4851.74 - R
Ax


Counter Clockwise (+)
M
Rcx
= 0
= R
Ax
(4.0in) W
t
( 2.0in)
R
Ax
=

= 2425.87 lbf
R
Cx
= 2425.87 = 2425.87 lbf
(Radial)
+ F
y
= 0
R
Cy
= 1765.89 - R
Ay

Counter Clockwise (+)

M
Rcy
= 0

= R
Ay
(4.0in) - W
r
(2.0in)

R
Ay
=

= 882.94 lbf

R
Cy
= 882.94 = 882.95 lbf

Tangential Shear & Bending Moment Calculations
From A to B:
+ F
x
= 0;
-R
Ax
V = 0
V = -R
Ax
= 429.109 lbf
M
o
= 0
R
Ax
(x) + M = 0
M = -R
Ax
(x)
M = 2425.87 (x)
From B to C:
+ F
x
= 0;
R
Ax
V - W
t
= 0
V= 2425.87-
V = 659.98 lbf
M
o
= 0
M - R
Ax
(x) + W
t
(x-2) = 0
M = +R
Ax
(x) +W
t
(x-2)
M = +2425.87 (x) + (x) 3531.78
M = -4191.76(x) 3531.78
Radial Shear & Bending Calculations
From A to B:
+ F
y
= 0;
-R
Ay
V = 0
V = -R
Ay
= - 882.94 lbf
M
o
= 0
R
Ay
(x) + M = 0
M = -R
Ay
(x)
M = - 882.94 (x)
From B to C:
+ F
y
= 0;
-R
Ay
V + W
r
= 0
V= - 882.94 + 1765.89
V = 882.95 lbf
M
o
= 0
M + R
Ay
(x) W
r
(x-2) = 0
M = -R
Ay
(x) +W
r
(x-2)
M = - 882.94 (x) + 1765.89 (x) 3531.78
M = 882.95(x) 3531.78























Basic Calculations for All Shaft Diameters
[

Shaft 1
Diameter 1(Input)
T=

Revised Cs No

, value remains the same Increased to easily machined value .625in

= .625 in

Diameter 2 (Bearing 1)

Increased to fit Bearing 6206 Size 1.1811 in

= 1.1811 in

Diameter 3 (Bearing 1 Shoulder)

Revised Cs=

= .912864

= 30503.23 psi
Recalculated

=Increased to fit Bearing 1 Shoulder .1.406 in

=1.406 in

Diameter 4 (Gear Diameter)

Revised Cs=

= .8216

= 29781.5 psi
Recalculated

Ring Groove Factor

= 1.70*1.06=.1.80 in

= Increased to easily machined value1.875 in

=1.875 in

Diameter 5 (Gear Shoulder)

Increased to make Gear Shoulder 2.0 in

= 2.0 in

Diameter 6 (Bearing 2 Shoulder)

Increased to fit Bearing 1 Shoulder Preferred = 1.406in

=1.406 in



Diameter 7 (Bearing 2)

Revised Cs

= .8943

= 33138 psi
Recalculated

= Increased to match Bearing 1 (6206) Size 1.1811 in

=1.1811 in


Shaft 2
Diameter 1 (Bearing 2)

Revised Cs

= .818119

= 32966.4 psi
Recalculated

= Increased to match Bearing 6207 Size 1.378 in

=1.378 in

Diameter 2 (Bearing 1 Shoulder)

Increased to fit Bearing 1 (6206) Shoulder Preferred = 1.406 in

= 1.406 in

Diameter 3 (Gear 1 Diameter)
T=

Revised Cs=

= .7997

= 29522.2 psi
Recalculated

Ring Groove Factor

*1.06 = 2.27 in
Final Value

= 2.27 in

Diameter 4 (Gear Shoulder)

= (

and

) increase larger diameter 0.23(in) to create gear shoulder 2.50 in

= 2.50 in


Diameter 5 (Gear 1 Diameter)

Revised Cs=

= .772

= 28348.9 psi
Recalculated

Ring Groove Factor

= 3.029*1.06 = 3.21089 in
Final Value

= 3.25 in

Diameter 6

Increased to fit Shoulder Preferred = 2.0 in

= 2.00 in

Diameter 7 (Bearing 2)

Revised Cs

= .8141

= 31304.91 psi
Recalculated

= Increased to match Bearing 2 (6209) Size 1.77 in

= 1.77 in

Shaft 3
Diameter 1 (Bearing 1)

Revised Cs

= .8456

= 31863.1 psi
Recalculated

Final Value

= 1.339 in
Diameter 2 (Bearing 1 Shoulder)

Increased to fit Bearing 1 Shoulder Preferred = 1.406in

= 1.406 in

Diameter 3 (Gear Shoulder)

Increased to make gear Shoulder 2.25 in

= 2.25 in

Diameter 4 (Gear Diameter)
T=

Revised Cs=

= .7912

= 28649.91 psi
Recalculated

Ring Groove Factor

=2.433*1.06= 2.579 in

= Increased to easily machined value2.6 in

=2.6 in

Diameter 5 (Bearing 2 Shoulder)

Revised Cs=

= .7912

= 29216.4 psi
Recalculated

(


)

Final Value

= 2.419 in

Diameter 6 (Bearing 2)

Increased to fit Bearing 6206 Size 1.1811 in

= 1.1811in

Diameter 7 (Output)

Revised Cs No

, value remains the same Increased to easily machined value 1.75in

= 1.75 in













Bearing Design Calculations
Shaft A
Bearing A

Bearing B

Shaft B
Bearing A

Bearing B

Shaft C
Bearing A

Bearing B

Keys
Gear A

= 0.01588

Gear B

= 0.06459

Gear C

= 0.06459

Gear D

= 0.03175