Beam Deflection Formulae
26 likes91,289 views
1. The document provides formulas for calculating slope, deflection, and maximum deflection for various beam types under different loading conditions. It gives the equations for cantilever beams with concentrated loads, uniformly distributed loads, and varying loads. It also provides the equations for simply supported beams with these different load types and with couple moments applied. The equations relate the beam properties like length, load location, and intensity to the resulting slope and deflection values.
More Related Content
What's hot (20)
Similar to Beam Deflection Formulae (20)
Recently uploaded (20)
Beam Deflection Formulae
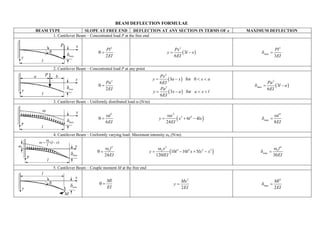
- 1. BEAM DEFLECTION FORMULAE BEAM TYPE SLOPE AT FREE END DEFLECTION AT ANY SECTION IN TERMS OF x MAXIMUM DEFLECTION 1. Cantilever Beam – Concentrated load P at the free end Pl 2 Px 2 Pl 3 θ= y= ( 3l − x ) δ max = 2 EI 6 EI 3EI 2. Cantilever Beam – Concentrated load P at any point Px 2 y= ( 3a − x ) for 0 < x < a Pa 2 6 EI Pa 2 θ= δ max = ( 3l − a ) 2 EI Pa 2 6 EI y= ( 3x − a ) for a < x < l 6 EI 3. Cantilever Beam – Uniformly distributed load ω (N/m) ωl 3 ωx 2 ωl 4 θ= 6 EI y= 24 EI ( x 2 + 6l 2 − 4lx ) δ max = 8 EI 4. Cantilever Beam – Uniformly varying load: Maximum intensity ωo (N/m) ωol 3 ωo x 2 ωo l 4 θ= 24 EI y= 120lEI (10l 3 − 10l 2 x + 5lx2 − x3 ) δ max = 30 EI 5. Cantilever Beam – Couple moment M at the free end Ml Mx 2 Ml 2 θ= y= δ max = EI 2 EI 2 EI
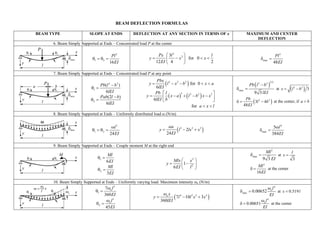
- 2. BEAM DEFLECTION FORMULAS BEAM TYPE SLOPE AT ENDS DEFLECTION AT ANY SECTION IN TERMS OF x MAXIMUM AND CENTER DEFLECTION 6. Beam Simply Supported at Ends – Concentrated load P at the center Pl 2 Px ⎛ 3l 2 ⎞ l Pl 3 θ1 = θ2 = y= ⎜ − x 2 ⎟ for 0 < x < δ max = 16 EI 12 EI ⎝ 4 ⎠ 2 48 EI 7. Beam Simply Supported at Ends – Concentrated load P at any point Pb(l 2 − b 2 ) y= Pbx 2 ( l − x2 − b2 ) for 0 < x < a Pb ( l 2 − b 2 ) 32 θ1 = 6lEI 6lEI δ max = 9 3 lEI at x = (l 2 − b2 ) 3 Pb ⎡ l 3⎤ ⎢ b ( x − a ) + (l − b ) x − x ⎥ 3 Pab(2l − b) y= 2 2 θ2 = 6lEI 6lEI ⎣ ⎦ δ= Pb 48 EI ( 3l 2 − 4b2 ) at the center, if a > b for a < x < l 8. Beam Simply Supported at Ends – Uniformly distributed load ω (N/m) ωl 3 ωx 3 5ωl 4 θ1 = θ2 = 24 EI y= 24 EI ( l − 2lx2 + x3 ) δmax = 384 EI 9. Beam Simply Supported at Ends – Couple moment M at the right end Ml 2 l Ml δmax = at x = θ1 = 9 3 EI 3 6 EI Mlx ⎛ x 2 ⎞ y= ⎜1 − ⎟ Ml 6 EI ⎝ l 2 ⎠ Ml 2 θ2 = δ= at the center 3EI 16 EI 10. Beam Simply Supported at Ends – Uniformly varying load: Maximum intensity ωo (N/m) 7ωol 3 ωo l 4 θ1 = δ max = 0.00652 at x = 0.519 l ωo x 360 EI ω l3 y= 360lEI ( 7l 4 − 10l 2 x 2 + 3x4 ) ωol 4 EI θ2 = o δ = 0.00651 at the center 45 EI EI